OPOD - 7 Supernumeraries
OPOD - 7 Supernumeraries: Exploring the Phenomenon of Multiple Fringes in Rainbows
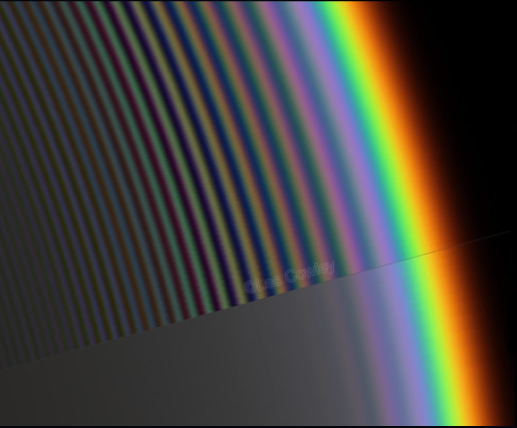
Rainbows have always fascinated us with their vibrant colors and ethereal beauty. But did you know that sometimes, rainbows can exhibit an even more extraordinary spectacle? In this article, we delve into the captivating phenomenon of supernumerary arcs or fringes in rainbows. While one or two fringes are commonly observed, it is exceptionally rare to witness a rainbow adorned with 7-8 of these mesmerizing arcs.
Supernumerary arcs appear as narrow, colored fringes situated near the inner purple edge of a primary rainbow. These striking features emerge when raindrops forming the bow are relatively small, measuring 1mm in diameter or less, and possess a uniform size. The spacing between the fringes widens as the drop size decreases. Consequently, the highest number of fringes is observed when the raindrops are uniformly sized, minimizing the blurring caused by overlapping fringe spacings. The existence of such a multitude of fringes implies that the raindrops responsible for this rainbow were remarkably uniform in size.
These supernumeraries are diffraction effects closely associated with a light caustic. As light waves are reflected once inside a raindrop, they form the primary rainbow. Upon emergence, these waves intersect and fold over each other, resulting in a pattern of crossing rays. The surface that distinguishes the region where rays intersect from the region without rays is called a caustic sheet. This fold caustic marks the intensely bright rim of the rainbow.
In close proximity to the caustic sheet, each intersecting pair of rays and waves coalesce and interfere with each other. When wave crests coincide, they create brightness, while out-of-phase crests produce darkness. This interference between coincident and out-of-phase wave crests gives rise to a set of light and dark diffraction fringes that run parallel to the caustic sheet. These fringes, known as supernumerary arcs, contribute to the awe-inspiring spectacle of the rainbow.
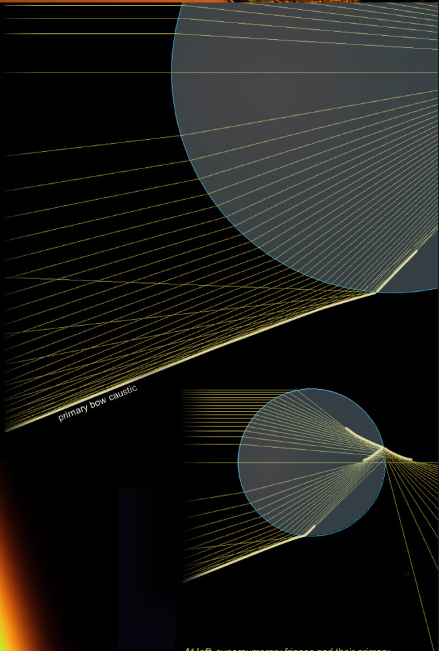
To understand the formation of supernumerary fringes, British Astronomer Royal George Biddell Airy developed a theory that assumes an algebraic form for the outgoing waves from the raindrop. Although this theory is approximate, it accurately simulates supernumeraries and is significantly faster than the later Mie-Lorentz theory, which is more computationally intensive but fully accurate. In the context of Airy's theory, the computation of supernumerary fringes involves considering a point source and raindrops with a uniform diameter of 0.7mm.
However, when raindrops have a spread of diameters or when a "real" sun with a diameter of 0.5° is taken into account, the intricate interference structure of supernumeraries becomes blurred to our eyes. These changes introduce variations in drop size and the size of the light source, impacting the clarity of the diffraction fringes.
In conclusion, the occurrence of multiple supernumerary arcs in rainbows is a captivating phenomenon that arises from the diffraction effects associated with a light caustic. The uniformity of raindrop size plays a crucial role in the visibility and abundance of these fringes. By understanding the underlying principles behind supernumeraries, we can appreciate the intricate beauty and complexity of atmospheric optics. So, keep your eyes peeled for these rare and enchanting rainbows, for they are a testament to the wonders of nature's light show.

Seven Supernumeraries
A remarkable rainbow imaged by Denis Betsch near Strasbourg, Alsace, France. ©Denis Betsch, shown with permission
Supernumerary arcs are narrow coloured fringes sometimes seen close to the inner purple edge of a primary rainbow. One or two fringes are a common sight but this rainbow with 7-8 arcs is rare indeed.
Supernumerary arcs or fringes are seen when the raindrops forming the bow are small (1mm dia. or less) and of uniform size. The fringe spacing widens with decreasing drop size and therefore most fringes are seen when the drops are of uniform size and there is less blurring from overlap of different fringe spacings. The drops must have been exceptionally uniform to produce so many fringes
Supernumeraries are diffraction effects associated with a light caustic.
Light waves reflected once inside a rain drop form the primary rainbow. They (strictly speaking waves rather than rays) emerge in a number of directions and as they do so they fold over and intersect each other. They form a pattern of crossing rays.
The surface separating the region of space occupied by two rays intersecting and that where there are no rays is a caustic sheet. The sheet, a fold caustic, marks the intensely bright rainbow rim.
Close to the caustic sheet, each intersecting ray/wave pair coalesces and interferes. Coincident wave crests give brightness, out of phase crests give darkness.
The result is a set of light and dark diffraction fringes parallel to the caustic sheet – These diffraction fringes are the supernumerary arcs.
At left, supernumerary fringes and their primary rainbow are computed using a theory developed by British Astronomer Royal George Biddell Airy (1801-1892). His theory makes assumptions about the algebraic form of the outgoing waves from the drop. Although it is approximate it is near enough to simulate supernumeraries and far quicker then the later, fully accurate but computationally laborious Mie-Lorentz theory.
The top slice shows supernumerary fringes from a point source and monosized drops 0.7mm diameter.
The lower slice is for of the same mean size but a spread of diameters plus a 'real' sun 0.5° diameter. Both changes blur the intricate supernumerary interference structure to our eyes.
Note: this article has been automatically converted from the old site and may not appear as intended. You can find the original article here.
Reference Atmospheric Optics
If you use any of the definitions, information, or data presented on Atmospheric Optics, please copy the link or reference below to properly credit us as the reference source. Thank you!
-
<a href="https://atoptics.co.uk/blog/opod-7-supernumeraries/">OPOD - 7 Supernumeraries</a>
-
"OPOD - 7 Supernumeraries". Atmospheric Optics. Accessed on April 18, 2024. https://atoptics.co.uk/blog/opod-7-supernumeraries/.
-
"OPOD - 7 Supernumeraries". Atmospheric Optics, https://atoptics.co.uk/blog/opod-7-supernumeraries/. Accessed 18 April, 2024
-
OPOD - 7 Supernumeraries. Atmospheric Optics. Retrieved from https://atoptics.co.uk/blog/opod-7-supernumeraries/.